Best posts made by MauriceMeilleur

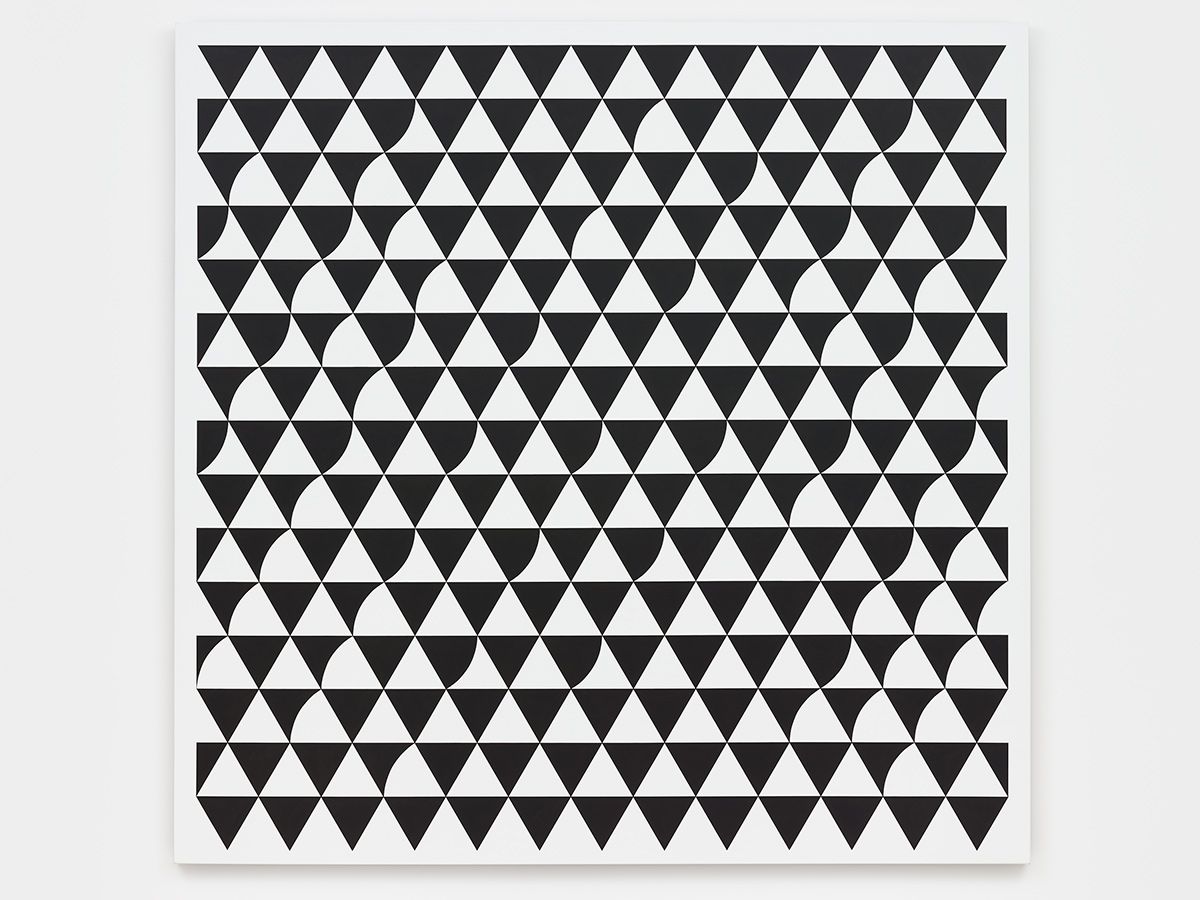
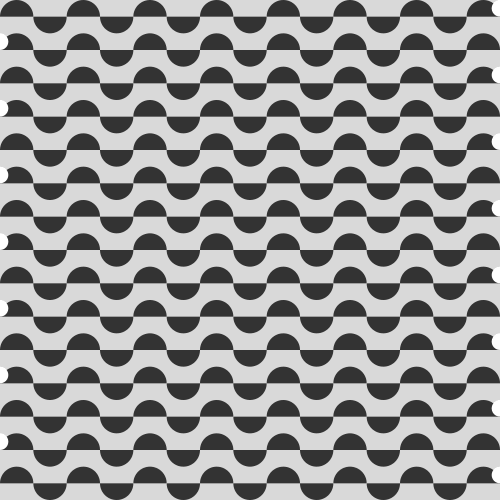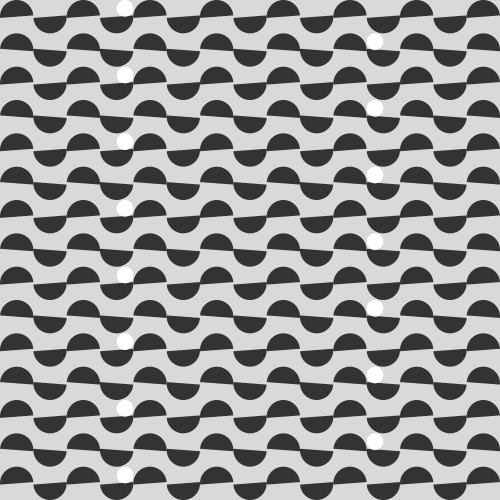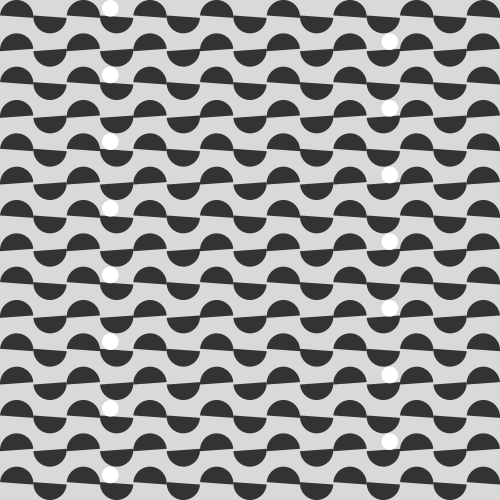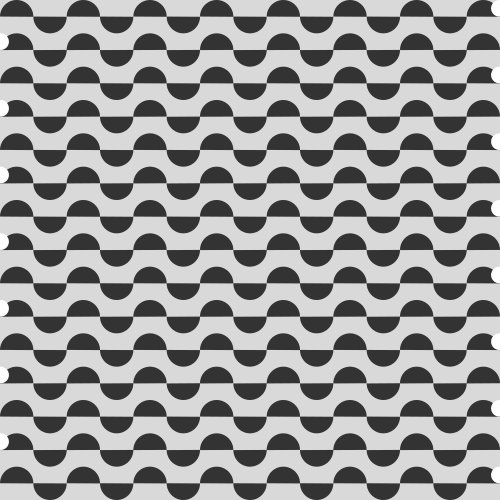
The finished product, a tribute to Bridget Riley's Rustle 6 (2015).

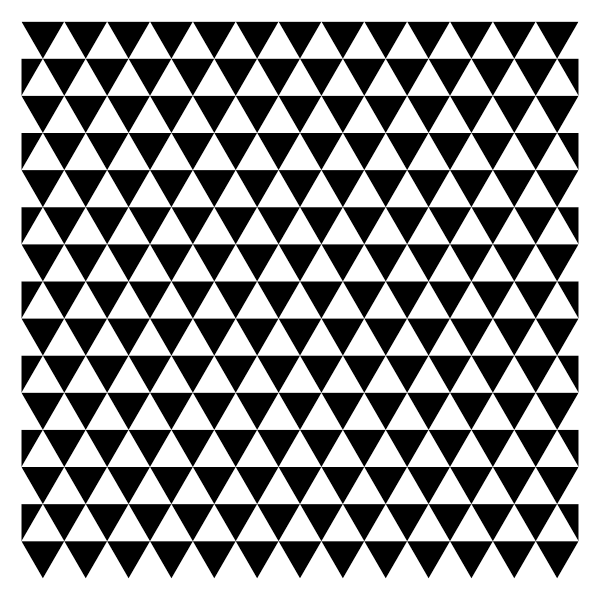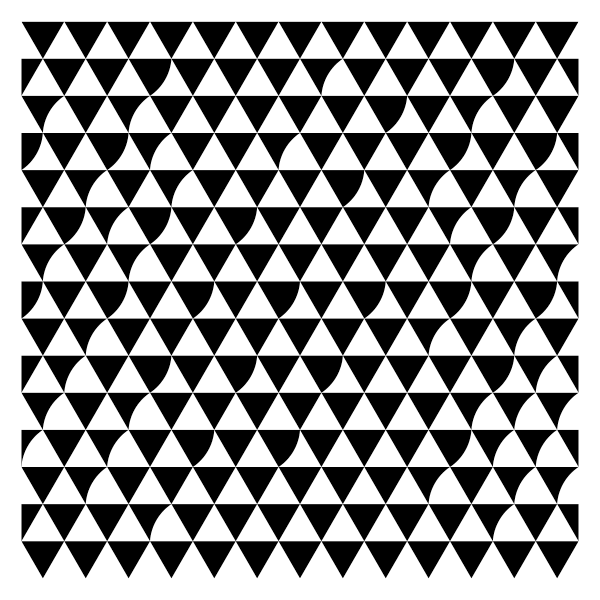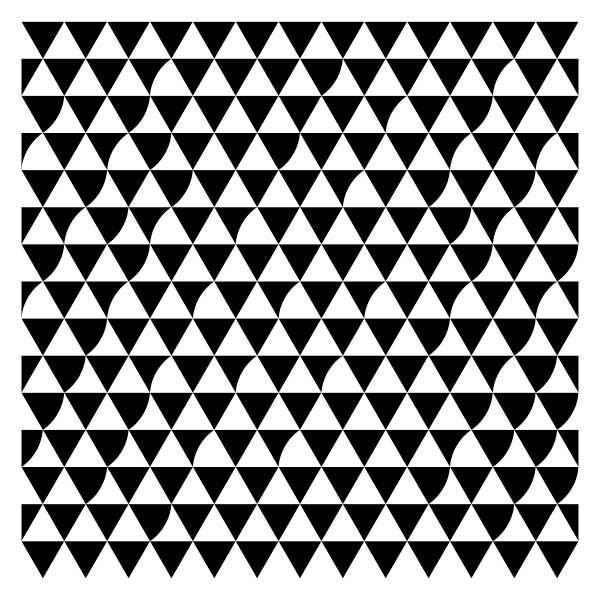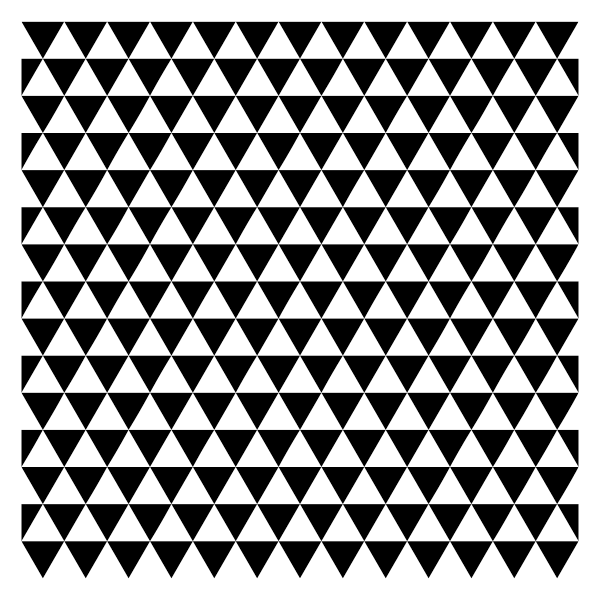
import math

canvasX = 600
canvasY = 600
nFrames = 120
side = canvasX/14
height = (side * sqrt(3))/2
center = (side/sqrt(3))/2
nX = 14
nY = 15
offsetX = side
offsetY = height

from datetime import *
time = datetime.now()

def interpolate(pt1, pt2, ratio):
    assert ratio <= 1
    return (pt1[0] + (ratio * (pt2[0] - pt1[0])), pt1[1] + (ratio * (pt2[1] - pt1[1])))
    
def triangle(s, h, c, inout):
    assert inout >= 0 and inout <= 1
    start = (-s/2, h/2)
    pivot = (0, h/2 - (c * 3))
    target = (s/2, h/2)
    control = interpolate((s/2, -c/2), (0, h/2 - c), inout)
    radius = s
    # optimal distance to control points is (4/3)*tan(pi/(2n); thank you https://stackoverflow.com/users/16673/suma for answering this question https://stackoverflow.com/questions/1734745/how-to-create-circle-with-b%C3%A9zier-curves
    control_ratio = ((4/3 * tan(pi/12)) * radius) / (2 * c)
    controlP = interpolate(pivot, control, control_ratio)
    controlT = interpolate(target, control, control_ratio)
    triangle = BezierPath()
    triangle.moveTo(start)
    triangle.lineTo(pivot)
    triangle.curveTo(controlP, controlT, target)
    triangle.closePath()
    drawPath(triangle)

def frame(x, y, m):
    f = BezierPath()
    g = BezierPath()
    f.rect(0, 0, x, y)
    g.rect(m, m, x - (2 * m), y - (2 * m))
    f = f.difference(g)
    f.closePath()
    drawPath(f)

# original Riley plan for Rustle 6    
plan = [
    [0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0],
    [0, 0, 0, 1, 0, 0, 0, -1, 0, 0, 0, 1, 0, 0],
    [-1, 0, -1, 0, 0, 0, 0, 0, 1, 0, -1, 0, 0],
    [1, 0, 1, -1, 0, 0, -1, 0, 0, 0, 1, 0, 1, 0],
    [0, -1, 0, -1, 0, 0, 0, 1, 0, -1, 0, -1, 0],
    [0, 1, -1, 1, 0, 1, 0, 0, 0, 0, -1, 1, 0, 0],
    [-1, 0, -1, 0, 0, 0, 0, 0, 0, 0, -1, 0, -1],
    [1, 0, 1, 0, 1, 0, 1, 0, 1, 0, 1, 0, 1, 0],
    [0, -1, 0, 0, 0, 0, 0, 0, 0, -1, 0, -1, 0],
    [0, -1, 0, 1, 0, 1, 0, 1, 0, 0, 0, 1, -1, 0],
    [-1, 0, -1, 0, 0, 0, 0, 0, 0, 0, -1, -1, -1],
    [-1, 0, -1, 0, 1, 0, 1, 0, 0, 0, 1, -1, -1, 0],
    [0, -1, 0, 0, 0, 0, 0, 0, 0, -1, 0, -1, -1, 0],
    [0, 0, 0, -1, 0, 0, 0, 0, 0, 0, 0, -1, 0, 0],
    [0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0, 0],
    ]

for n in range(nFrames):
    newPage(canvasX, canvasY)
    frameDuration(1/30)
    ex = sin(radians(n * (360/nFrames)))
    fill(1)
    rect(0, 0, canvasX, canvasY)
    with savedState():
        translate(canvasX/2, canvasY/2)
        fill(0)
        translate(-(nX - 1) * offsetX/2, (nY - 1) * offsetY/2)
        for i in range(nY):
            with savedState():
                translate(0, i * -offsetY)
                if i % 2 == 0:
                    for j in range(nX - 1):
                        with savedState():
                            translate(offsetX/2 + (j * offsetX), 0)
                            triangle(side, height, center, .5 - (ex * (plan[i][j] * .5)))
                else:
                    for j in range(nX):
                        with savedState():
                            translate(j * offsetX, 0)
                            triangle(side, height, center, .5 - (ex * (plan[i][j] * .5)))
    fill(1)
    frame(canvasX, canvasY, side/2)

saveImage('~/Desktop/riley_tribute_test_01_%s.gif' %time)

The reference image:

I mean to do so at some point—probably on GitHub, probably this summer when I can take a little time to clean up code and organize the sketches.

And here's yet another—I forget the name of the illusion just now.

canvas = 500 #750
number = 15
shape = canvas/15
small = shape/2
offset = shape
nFrames = 90 #240
r = 5

def semi(pos, rot):
    s = BezierPath()
    if pos == 'top':
        s.arc((0, 0), shape/2, 0, 180, False)
    elif pos == 'bottom':
        s.arc((0, 0), shape/2, 0, 180, True)
    s.closePath()
    s.rotate(rot)
    drawPath(s)
    
for n in range(nFrames):
    newPage(canvas, canvas)
    frameDuration(1/30)
    fill(.85)
    rect(0, 0, canvas, canvas)
    translate(canvas/2, canvas/2)
    save()
    translate(-(number - 1) * offset/2, (number - 1) * offset/2)
    fill(.2)
    for i in range(number):
        save()
        translate(0, i * -offset)
        if (i % 2) == 0:
            v = 1
        elif (i % 2) == 1:
            v = -1
        for j in range(number):
            save()
            translate(j * offset, 0)
            if (j % 2) == 0:
                semi('top', r * (sin((2 * pi) * (n/nFrames))) * v)
            elif (j % 2) == 1:
                semi('bottom', r * (sin((2 * pi) * (n/nFrames))) * v)
            restore()
        restore()
    restore()
    translate(0, (number - 1) * offset/2)
    for i in range(number):
        save()
        translate(0, i * -offset)
        if (i % 2) == 0:
            fill(1)
            oval((cos((2 * pi) * (n/nFrames)) * canvas/2) - small/2, 0, small, small)
        elif (i % 2) == 1:
            fill(1)
            oval((cos((2 * pi) * (n/nFrames)) * -canvas/2) - small/2, 0, small, small)
        restore()
saveImage('~/Desktop/op_semi_lines.gif')

With both sketches, a larger canvas and more frames (240+) makes for a better effect.

Here's code for another, the 'intertwining illusion'.

canvas = 500
nFrames = 60 #240
size = canvas/36
num1 = 60
num2 = 44
num3 = 30
num4 = 14
r = 15

def mod(rot):
    m = BezierPath()
    m.rect(-size/2, -size/2, size, size)
    m.closePath()
    m.rotate(rot)
    drawPath(m)
    
for n in range(nFrames):
    newPage(canvas, canvas)
    frameDuration(1/30)
    fill(.5)
    rect(0, 0, canvas, canvas)
    strokeWidth(3)
    translate(canvas/2, canvas/2)
    save()
    for i in range(num1):
        if i % 2 == 0:
            stroke(1)
        else:
            stroke(.15)
        save()
        translate(.8 * canvas/2, 0)
        mod(r * (sin(2 * pi * n/nFrames)))
        restore()
        rotate(360/num1)
    restore()
    save()
    for j in range(num2):
        if j % 2 == 0:
            stroke(1)
        else:
            stroke(.15)
        save()
        translate(.6 * canvas/2, 0)
        mod(-r * (sin(2 * pi * n/nFrames)))
        restore()
        rotate(360/num2)    
    restore()
    save()
    for k in range(num3):
        if k % 2 == 0:
            stroke(1)
        else:
            stroke(.15)
        save()
        translate(.4 * canvas/2, 0)
        mod(r * (sin(2 * pi * n/nFrames)))
        restore()
        rotate(360/num3)    
    restore()
    save()
    for l in range(num4):
        if l % 2 == 0:
            stroke(1)
        else:
            stroke(.15)
        save()
        translate(.2 * canvas/2, 0)
        mod(-r * (sin(2 * pi * n/nFrames)))
        restore()
        rotate(360/num4)    
    restore()

saveImage('~/Desktop/opart_intertwine.gif')

The more frames, the more subtle the shift.

Good news about the pen functionality—glad I saw that, it'll come in handy for a couple things I'm working on …

@michelangelo Ned, please feel free to email me at maurice@mauricemeilleur.net. This is a bit of a specialty of mine.